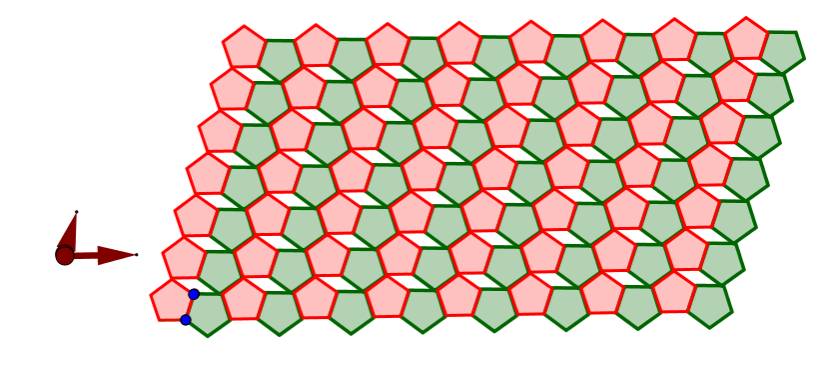
Exploring Regular Edge-Edge Tessellations of the Cartesian Plane and the Mathematics behind it
Mathematics and Art Activity - Basic Plane Tessellations with GeoGebra
Goal: To enable Maths educators to use GeoGebra to understand some of the mathematics that supports the construction of regular plane tessellations.
Relevant Maths Keywords and Concepts: Tessellation or Tiling, Euclidean Plane, Regular shape, Irregular shape, Regular Polygon, Interior angles, Exterior angles, Congruent shapes, Regular Tessellation, Irregular Tessellation
1. Pre-Knowledge for exploring basic plane tessellations:Polygons are 2-dimensional shapes. They are made of straight lines (edges), and the shape is "closed" (all the lines connect up in vertices).Regular Polygons are polygons that are equiangular (all angles are equal in measure) and equilateral (all sides have the same length).You should also know that:
· a whole turn around any point on a surface is 360°
· the sum of the internal angles of any triangle = 180°
· the sum of the internal angles of any quadrilateral = 360°
· the sum of the external angles of any polygon=360° (one whole turn)
· the sum of the interior angles of a n-sided regular polygon = (n -2) × 180°
· how to calculate or measure the interior angles of regular polygons
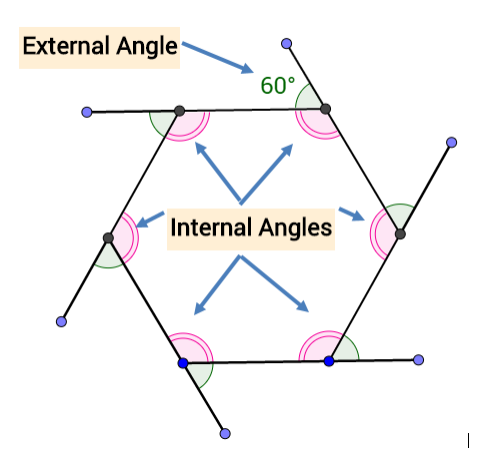
Internal and External Angles of a Regular Polygon
Example:The sum of the internal angles of a regular Hexagon (n=6) is (n - 2) × 180°=(6 - 2) × 180°=720°.Hence the internal angles of a regular Hexagon is 720°/6= 120°.
Symmetry
Symmetry is the property that a figure coincides with itself under an isometry, where an isometry is an action (movement) in the plane that preserves size and shape. There are three basic types of isometries that present symmetry of a figure in a plane.
Types of Symmetry:
(a) Reflectional symmetry. An object has reflectional symmetry if you can reflect it in a way such that the resulting image coincides with the original. Hold a mirror up to it, its reflection looks identical.
(b) Rotational symmetry. An object has rotational symmetry if it can be rotated about a point in such a way that its rotated image coincides with the original figure before turning a full 360°.
(c) Translational symmetry. An object has translational symmetry if you move it along a straight path without turning it to produce the same image.
Defining a Tessellation and a Regular Tessellation of a plane
A tessellation can be defined as the covering of a plane with a repeating unit consisting of one or more shapes (regular or irregular) in such a way that: • there are no open spaces between and no overlapping of the shapes that are used;• the covering process has the potential to continue indefinitely (for a surface of infinite dimensions– Cartesian Plane).
Regular Tessellations of the Plane
Tessellations in which one regular polygon is used repeatedly are called regular tessellations.
Two key questions to consider – Which regular polygons will tessellate (or tile) the plane and why?How many different tessellations are possible in each case?
Naming convention for Regular Plane Tessellations
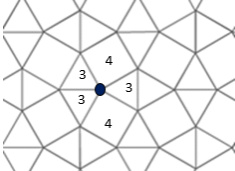
Consider the example of an edge-edge plane tessellation in Figure 1. Although all the polygons are regular, there are more than one type of polygon which that are used to tessellate. This makes this a non-regular tessellation (or tiling) of the plane.
A vertex is a common point where sides (edges) of polygons meet. The configuration of a vertex is the sequence of polygon orders that exist around it. Normally these orders are given in a sequence starting with the lowest order. The vertex configuration of each vertex in the tiling shown in Figure 1. is 3.3.4.3.4 as each vertex is surrounded by two equilateral triangles, a square, another equilateral triangle and finally a square.
Clearly the vertex configuration of each vertex of a regular tessellations of the plane will be identical.
Figure 1.
Only Three Regular Edge-Edge Plane Tessellations Exist
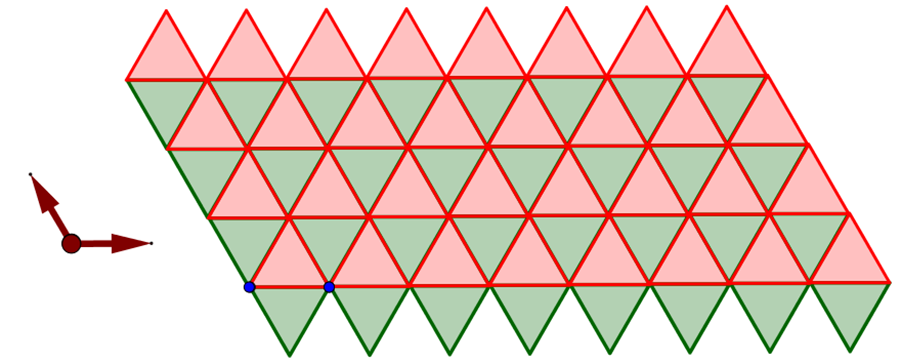
Equilateral Triangle 3.3.3.3.3.3 Tiling
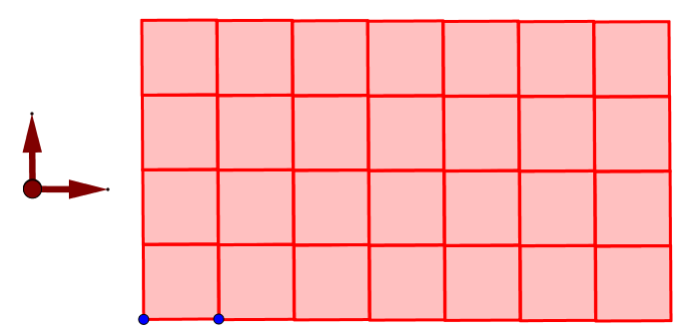
Square 4.4.4.4 Tiling
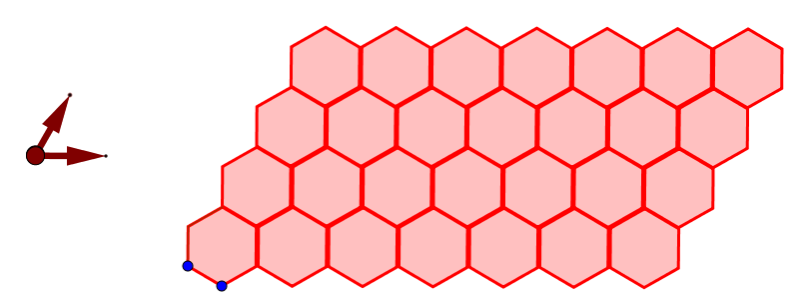
Hexagon 6.6.6 Tiling
No other regular polygon will tile the plane as their inner angles are not a factor of 360°.
See for instance a Pentagon:
Pentagons does not tessellate the plane - open uncovered spaces will always exist.
