Google Classroom
GeoGebraGeoGebra Classroom
GeoGebra

Finding the Median of a Trapezoid

Author:Sarjarbinx17, GeoGebra Materials Team, Irena Štrausová
Topic:Geometry

Analyze the dimensions of the trapezoid around and use the slider to create a parallelogram.

Use the slider to transform the trapezoid into a parallelogram. Observe the different dimensions/measurements of the parallelogram and the trapezoid and compare their shapes and properties. Which of the following properties do trapezoids possess that are different from parallelograms?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

Use the slider to transform the trapezoid into a parallelogram. Base 1 has a length of 12 inches, Base 2 has a length of 4 inches, and the height of the trapezoid is 7 inches. Use your knowledge of calculating the area of a parallelogram to calculate the area of the trapezoid. What is the area of the trapezoid?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

Area of Trapezoid

Area of Trapezoid
Based off of calculating the area of a parallelogram and dividing that into two trapezoids, we can see that the formula for finding the area of a trapezoid is 1/2 that of a parallelogram. Therefore, the area of a trapezoid is 1/2h(a+b).

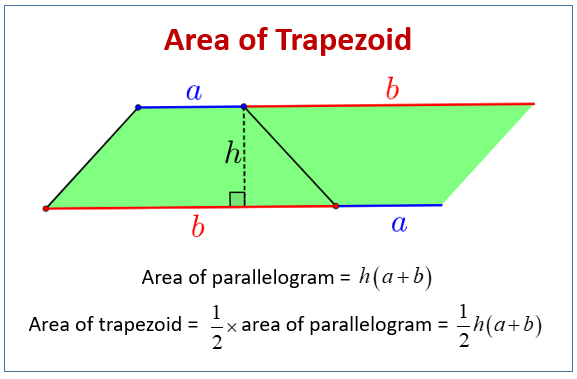
Analyze this trapezoid without sliding the red line. In this instance, c is Base 1 and a is Base 2. Using what you now know about the formula for calculating the areas of trapezoids and parallelograms, hypothesize the formula used to find the midpoint (also know as the median) of this trapezoid.

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)
Now use the slider to move the red point. As we can see by reconstructing and manipulating the trapezoid into a rectangle, the formula used to calculate the median of a a trapezoid is (base 1+base 2)/2).
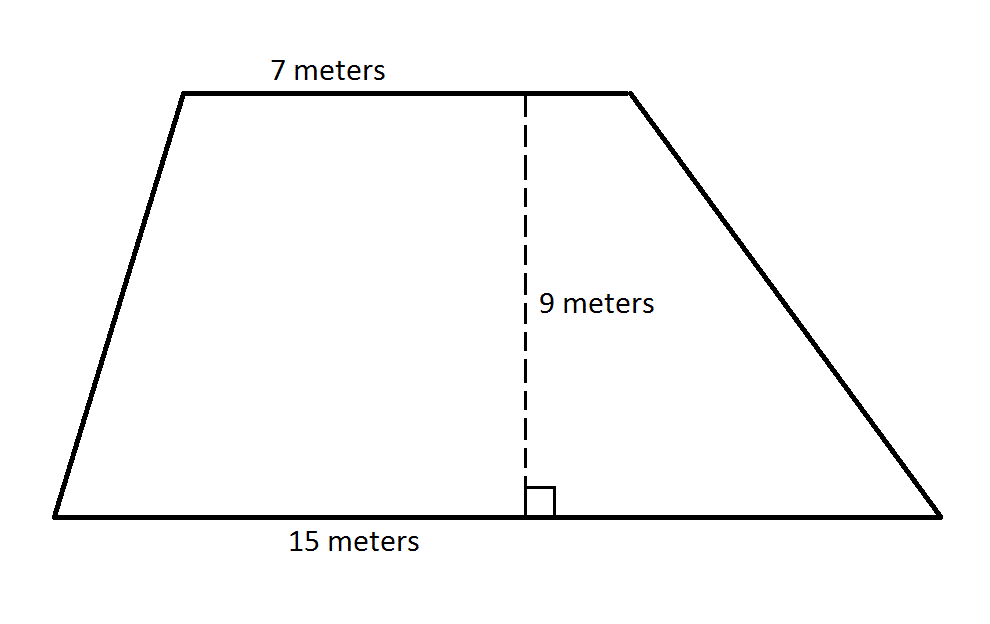
Image

Observe this trapezoid. Now with the knowledge of calculating trapezoid medians, calculate the median for this specific trapezoid given the allotted dimensions and measurements.

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

New Resources

Discover Resources

Discover Topics