Adding the first n squares
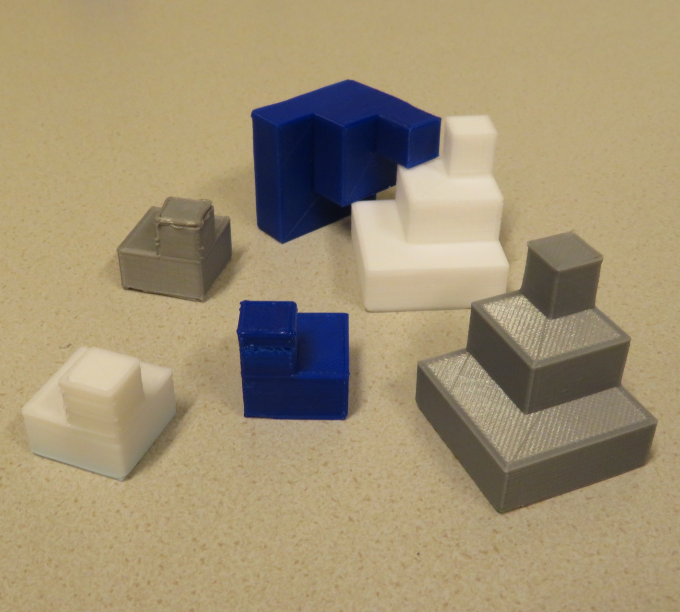
In this applet, we use 3D blocks to represent an important identity of Number Theory.
adding squares - piece 2
note: you can directly open this material in GeoGebra 3D Graphing just clicking in https://www.geogebra.org/3d/pe9wgzjs
adding squares - piece 3
note: you can directly open this material in GeoGebra 3D Graphing just clicking in https://www.geogebra.org/3d/u332rret
It is well known that Also well known, but more difficult to prove that Usually this latter formula is proven by using induction, but it hides the geometrical background of the right side of the equation.
Recently I read a "proof without words" explanation of this important formula, published by Man-Keung Siu from the University of Hong Kong.
The applet is limited to for technical reasons. Although, if you download the material, in the desktop version you may want to increase . Nevertheless, the proof is still easy to understand even if . For all cases, you have to consider the height of each level is 1.
Actually, the proven formula is , which is equivalent to the previous one.