Google Classroom
GeoGebraGeoGebra Classroom
GeoGebra

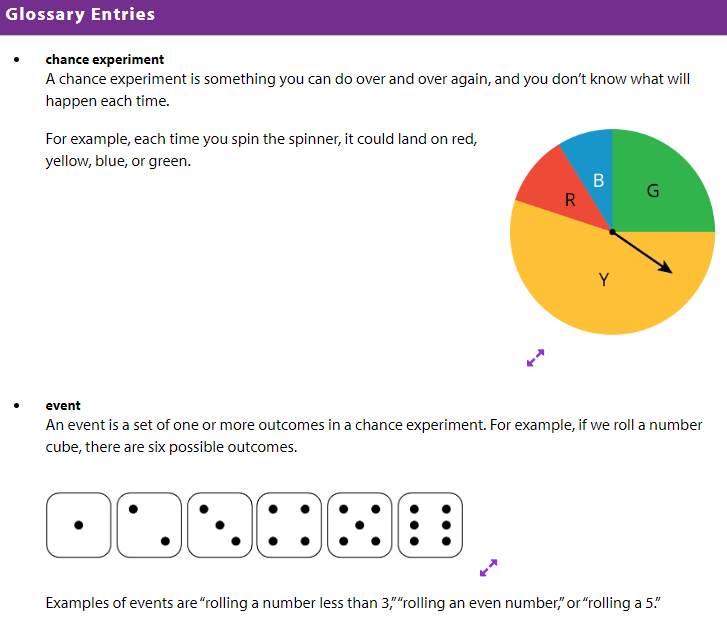
L8.4 - Tables of Relative Frequencies

Autore:Ms. Leon-Guerrero (Ms. LG)
Argomento:Probabilità

Learning Intentions and Success Criteria

We are learning to:
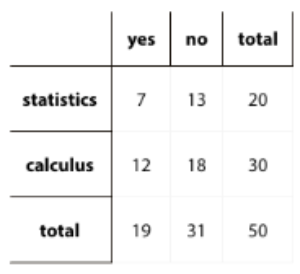
  • Interpret a two-way table that represents a sample space
  • Use information in a two-way table to calculate relative frequencies and to estimate probabilities
We are successful when we can:
  • Use information in a two-way table to find relative frequencies and to estimate probability
Approximately symbol ≈
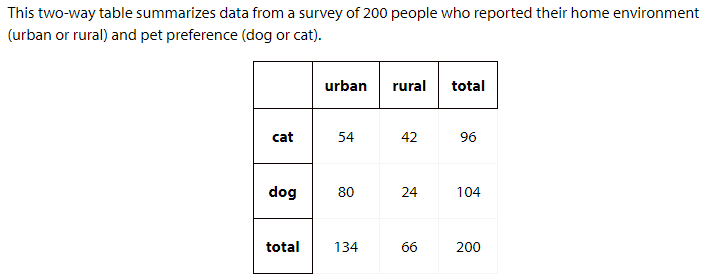
Image
Image

What do you notice? What do you wonder?

4.2: Rolling into Tables

Open Geo.8.4 Tables of Relative Frequencies, scoll down to 4.2: Rolling into Tables, follow instructions and record your results in the Jamboard L8.4 - Tables of Relative Frequencies.

1. Do the values in the table match your expectation? Explain your reasoning.

2. Based on the table, how many times did partner A roll a 5?

3. How many times did you both roll the same number?

4. What percentage of the rolls resulted in the same number from both partners?

5. What percentage of the rolls resulted in partner A rolling a 3 and partner B rolling a 6?

6. Based on the table, estimate the probability that partner A will roll a 2 and partner B will roll a 4. Explain your reasoning.

4.3: Traveling Methods

Data A: A company has an office in Austin, Texas, and an office in Copenhagen, Denmark. The company wants to know how employees get to work, so they take a survey of all the employees and summarize the results in a table.
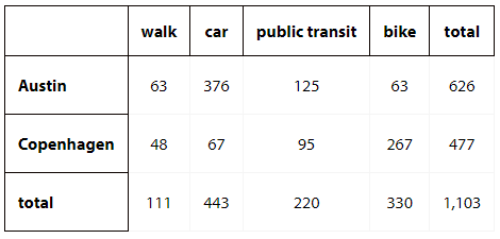
Image

1. If an employee is selected at random, what is the probability that they work in Austin and drive a car to work?

2. If an employee is selected at random, what is the probability that they work in Copenhagen and ride a bike to work?

3. If an employee is selected at random, what is the probability that they take public transit to work?

4. If an employee from Copenhagen is selected at random, what is the probability that they ride a bike to work?

5. If an employee who takes public transit to work is selected at random, what is the probability they work in Austin?

6. How are the last two questions different from the first three?

Data B: A school district is interested in how students get to school, so they survey their high school students to see how they get to school and separate the numbers by grade level. The results of the survey are summarized in the table.
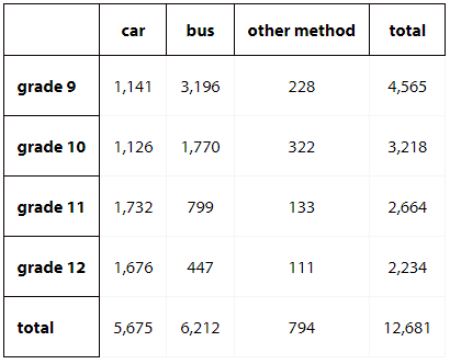
Image

1. If a high school student is selected at random, what is the probability they are in grade 9 and ride the bus to school?

2. If a high school student is selected at random, what is the probability that they are in grade 12?

3. If a high school student is selected at random, what is the probability that they take a car to school?

4. If a grade 10 student is selected at random, what is the probability that they ride a bus to school?

5. If a grade 12 student is selected at random, what is the probability that they ride a bus to school?

6. If a student who rides the bus to school is selected at random, what is the probability that they are in grade 9?

Lesson Synthesis: Tables of Relative Frequencies

This table shows the responses to a survey question, “Do you like homework?”
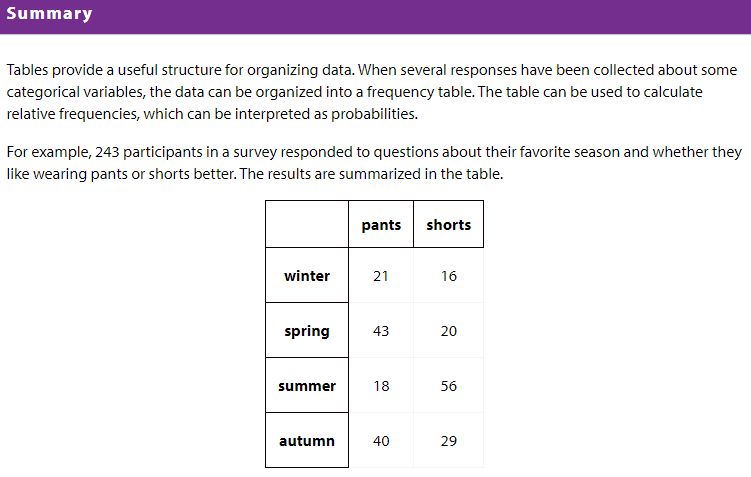
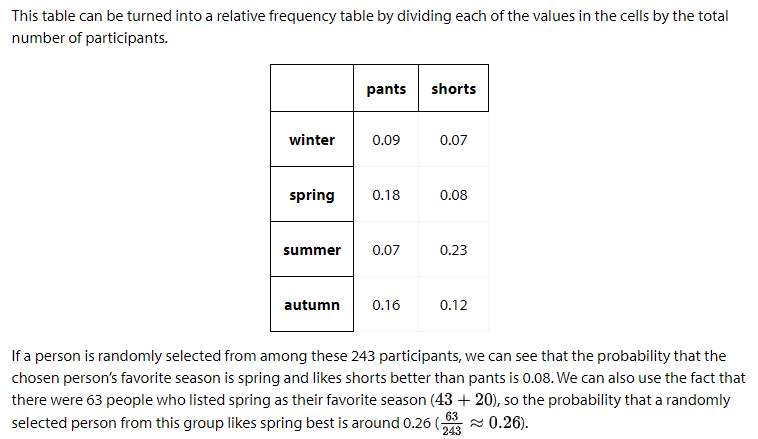
Image
Image
Image
Image

Learning Intentions and Success Criteria

We are learning to:
  • Interpret a two-way table that represents a sample space
  • Use information in a two-way table to calculate relative frequencies and to estimate probabilities
We are successful when we can:
  • Use information in a two-way table to find relative frequencies and to estimate probability

Cool-Down: School Attendance

A district is interested in attendance for students in middle and high school. The results for one day are summarized in the table.
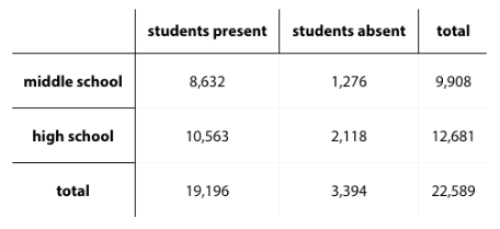
Image

1. If a student’s name is selected at random, what is the probability that they are a middle school student who was present?

2. If high school student’s name is selected at random, what is the probability that they were absent on that day?

3. If a student who was present that day is selected at random, what is the probability that they are in middle school?

Image
Image

Nuove risorse

Scopri le risorse

Scopri gli argomenti