Slope, Linear Whole Day Lesson
Lesson 4Problem 1A contractor must haul a large amount of dirt to a work site. She collected information from two hauling companies. EZ Excavation gives its prices in a table. Happy Hauling Service gives its prices in a graph. dirt (cubic yards)cost (dollars)row 1row 2row 3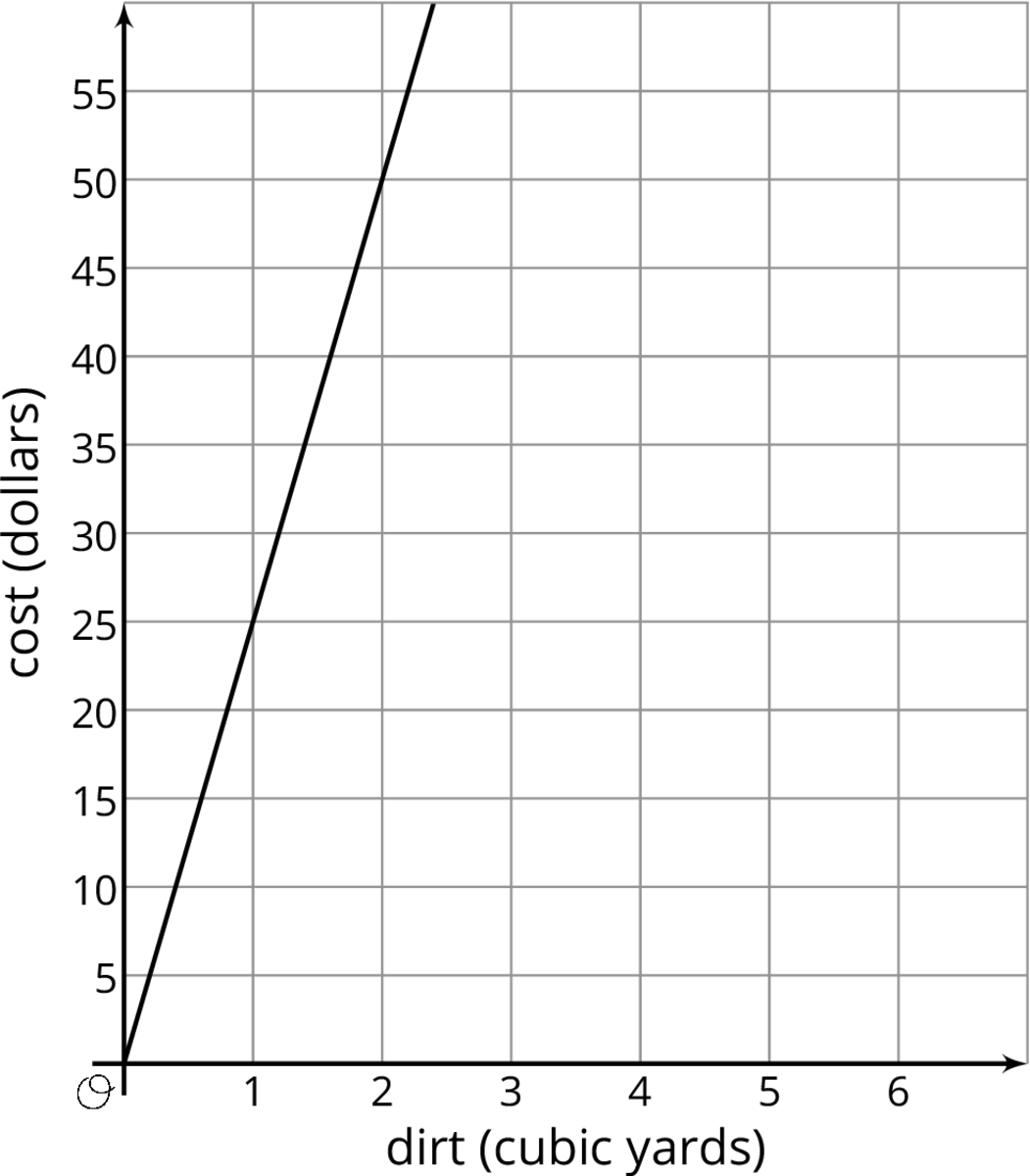
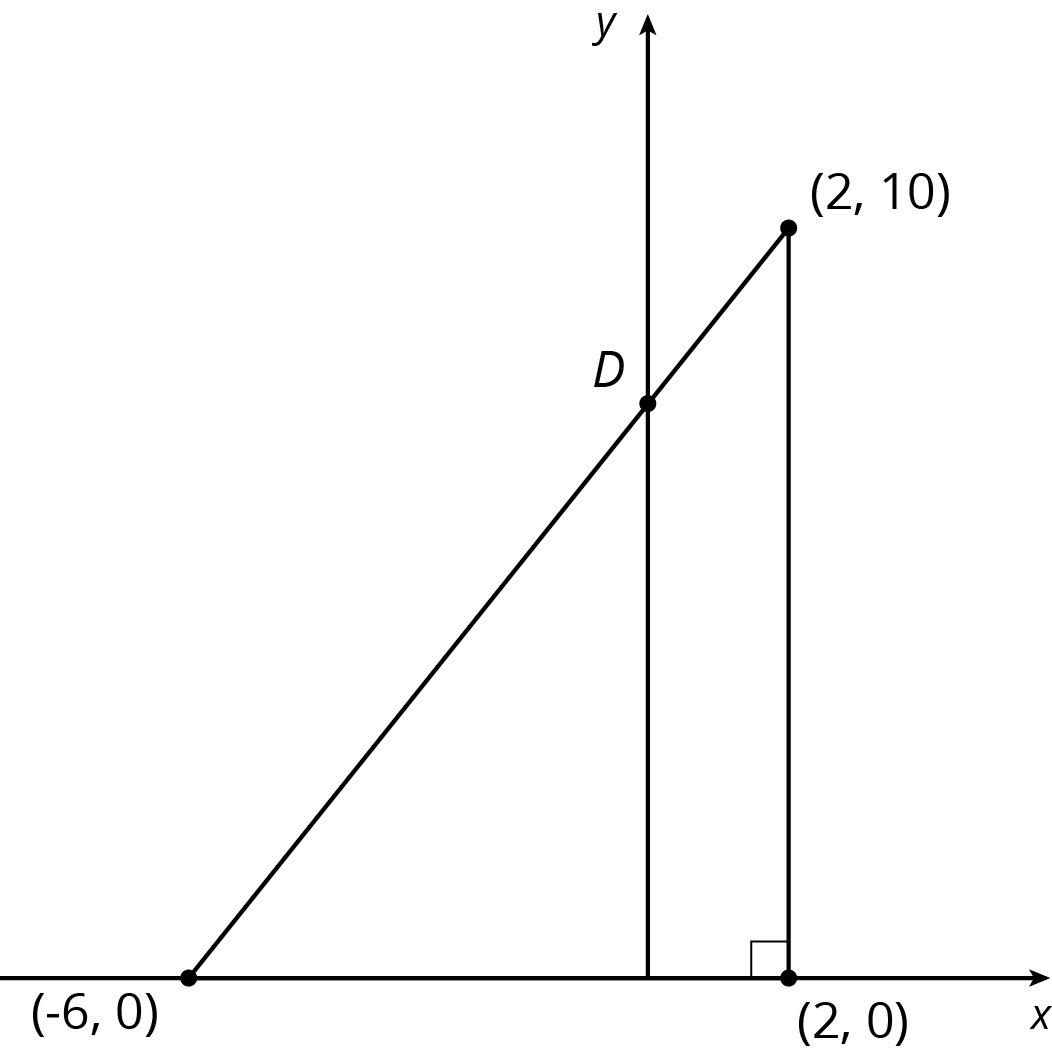
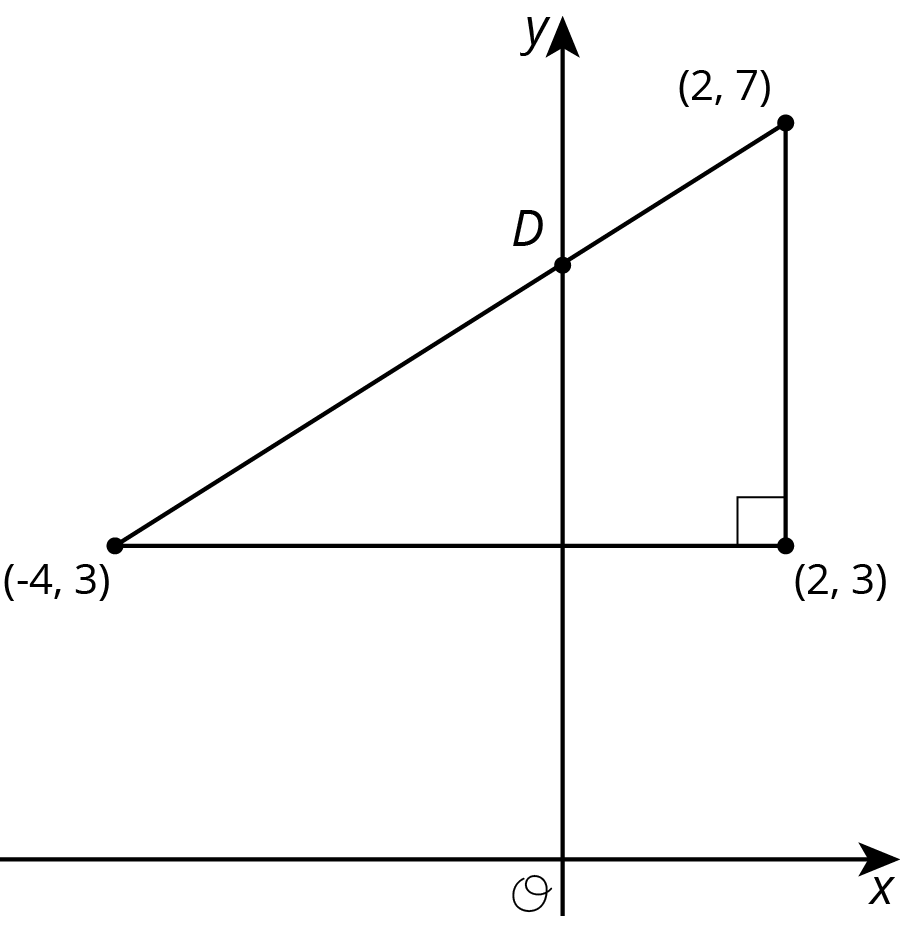 Problem 4 (from Unit 2, Lesson 11)Select all the pairs of points so that the line between those points has slope 23.
Problem 4 (from Unit 2, Lesson 11)Select all the pairs of points so that the line between those points has slope 23.
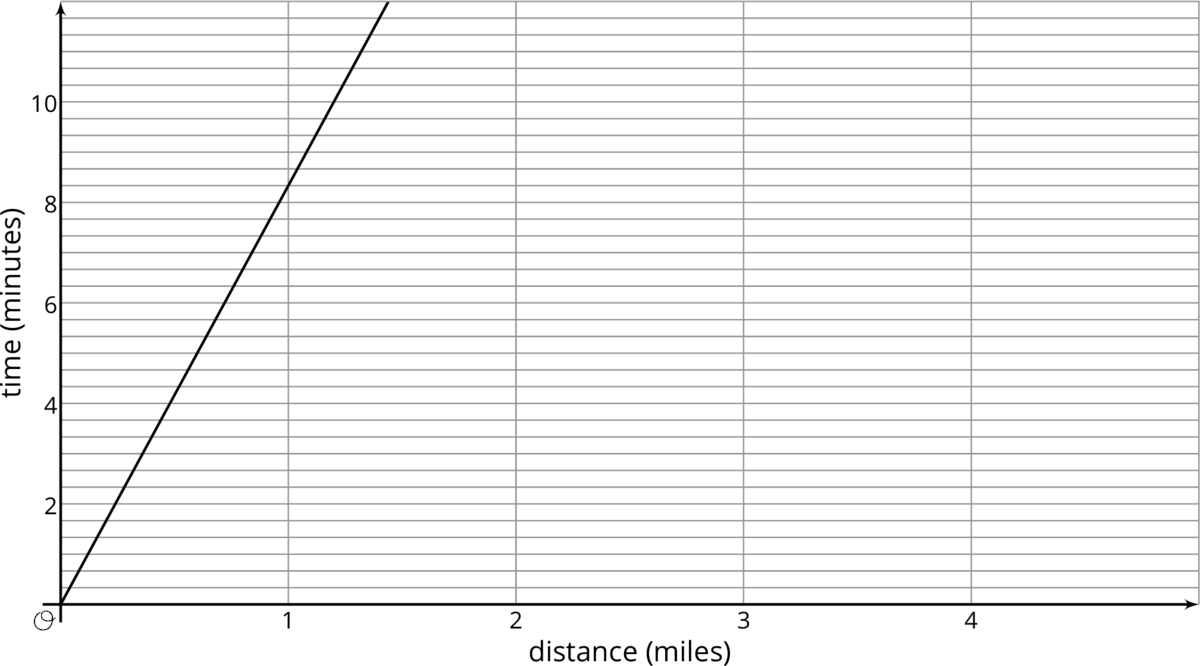 Elena’s distances and times for a training run are given by the equation y=8.5x, where x represents distance in miles and yrepresents time in minutes.
Elena’s distances and times for a training run are given by the equation y=8.5x, where x represents distance in miles and yrepresents time in minutes.

- How much would each hauling company charge to haul 40 cubic yards of dirt? Explain or show your reasoning.
- Calculate the rate of change for each relationship. What do they mean for each company?
- If the contractor has 40 cubic yards of dirt to haul and a budget of $1000, which hauling company should she hire? Explain or show your reasoning.

 Problem 4 (from Unit 2, Lesson 11)Select all the pairs of points so that the line between those points has slope 23.
Problem 4 (from Unit 2, Lesson 11)Select all the pairs of points so that the line between those points has slope 23.- (0,0) and (2,3)
- (0,0) and (3,2)
- (1,5) and (4,7)
- (-2,-2) and (4,2)
- (20,30) and (-20,-30)

- How much would the painting company charge to paint a house that needs 20 hours of labor? A house that needs 50 hours?
- Draw a line representing the relationship between x, the number of hours it takes the painting company to finish the house, and y, the total cost of painting the house. Label the two points from the earlier question on your graph.
- Find the slope of the line. What is the meaning of the slope in this context?
 Elena’s distances and times for a training run are given by the equation y=8.5x, where x represents distance in miles and yrepresents time in minutes.
Elena’s distances and times for a training run are given by the equation y=8.5x, where x represents distance in miles and yrepresents time in minutes.- Who ran farther in 10 minutes? How much farther? Explain how you know.
- Calculate each runner's pace in minutes per mile.
- Who ran faster during the training run? Explain or show your reasoning.
Review
Assesment/Exit Ticket
- For each graph, record:vertical changehorizontal changeslope
- Describe a procedure for finding the slope between any two points on a line.
- Write an expression for the slope of the line in the graph using the letters u,v,s,u,v,s, and tt.