Parallelograms: IM Lesson 6.1.4
This GeoGebra activity has been created from this lesson authored by Illustrative Mathematics.
Figures A, B, and C are parallelograms. Figures D, E, and F are not parallelograms.
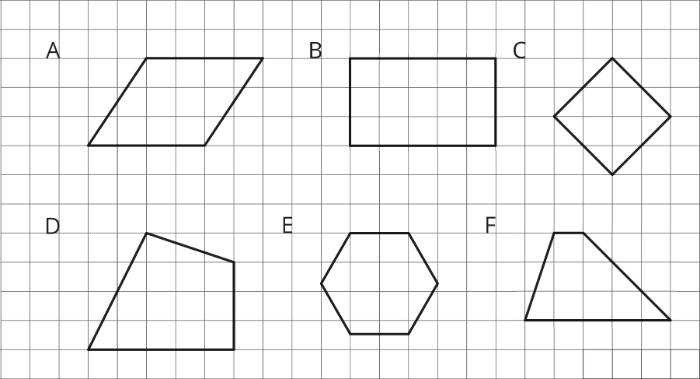
Study the figures above.
How many sides does a parallelogram have?
Do you notice anything about opposite angles of a parallelogram? If so, describe.
Do you notice anything about opposite sides of a parallelogram? If so, describe.
An empty parallelogram is shown on the left. Use any 1 or more of the colored polygons to help find its area.
What is the area of the parallelogram above? Explain your reasoning.
Change the parallelogram by dragging the green points at its vertices. Then use any 1 or more of the colored polygons to help find its area.
What is the area of your parallelogram now? Explain your reasoning.
If you used the polygons on the side, how were they helpful? If you did not, try using one or more of the polygons to show another way to find the area of the parallelogram in the app above.
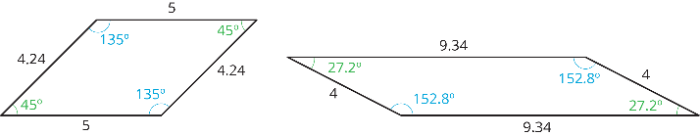
Use the parallelograms shown in A and B to help answer the questions below.
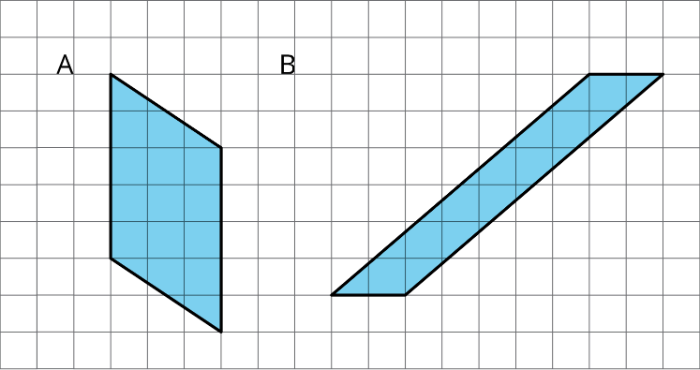
What is the area of parallelogram A? Explain your thought process.
What is the area of parallelogram B? Explain your reasoning.
For the question below, refer to parallelogram C shown here.
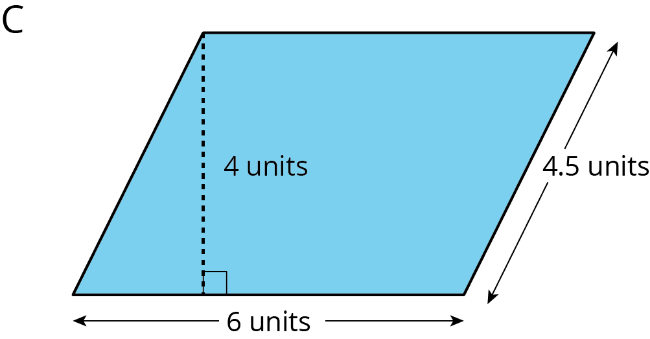
What is the area of parallelogram C? Explain how you know.
SUMMARY
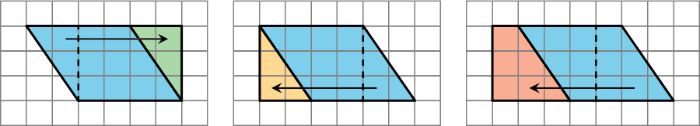
There are several strategies for finding the area of a parallelogram:
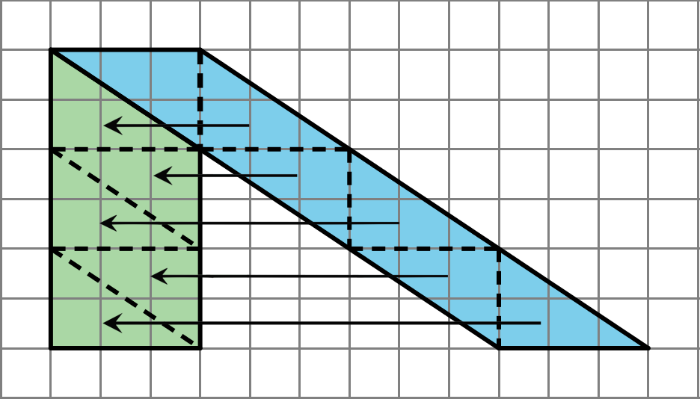
We can decompose and rearrange a parallelogram to form a rectangle. Here are three ways:
We can enclose the parallelogram and then subtract the areas of the two triangles in the corner.
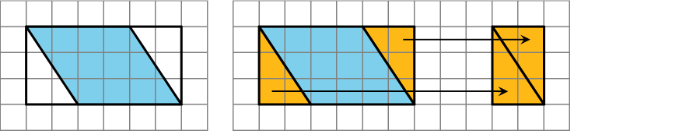
Both of these ways will work for any parallelogram. However, for some parallelograms the process of decomposing and rearranging requires a lot more steps than if we enclose the parallelogram with a rectangle and subtract the combined area of the two trian