Gonbad-e Sork
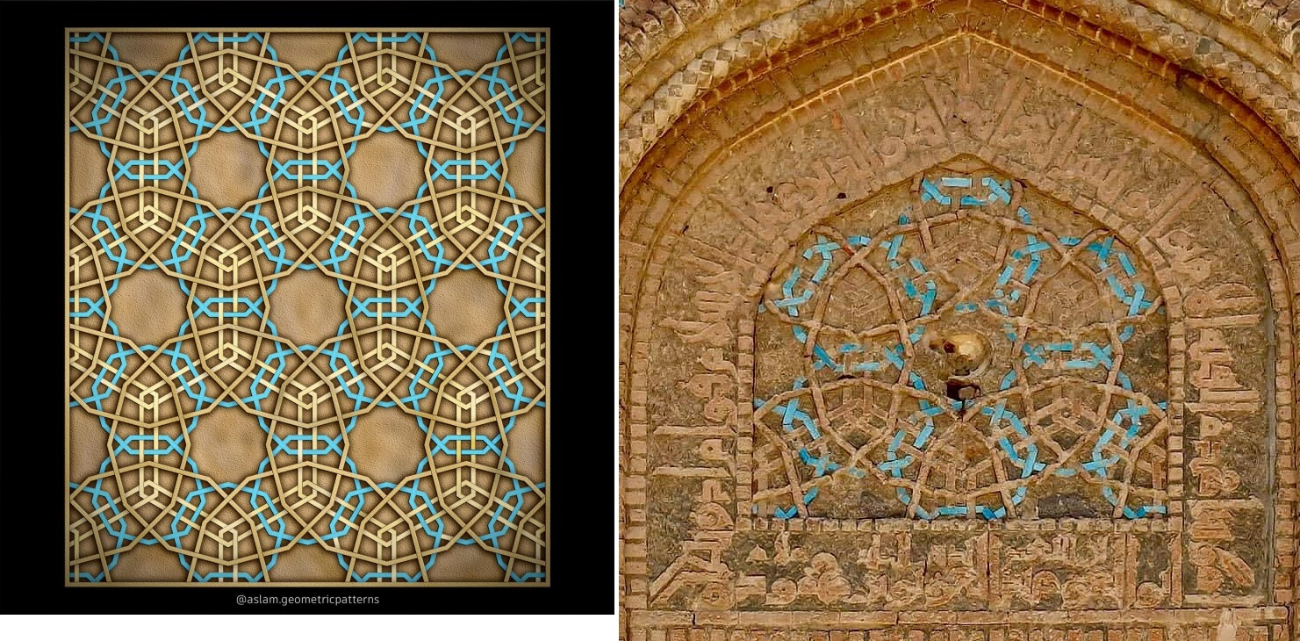
The Gonbad-e Sork, a square building with accentuated columns at the corners, is het oldest of the 5 still remaining tomb towers in Maragha. The building date 1148 is written on the tympan, together with the name of the architect. The shrine is the earliest Iranian monument in which glazed bricks appear in the decoration. Sidewalls and columns at the corners are decorated with different patterns, but most remarkable is the tympan with a complicated line pattern.

Superposed are three patterns around one common point.
- Intertwined irregular nonagons are made out of glazed turquoise strips.
- A second pattern is made out of intertwined hexagons, each with a 6-pointed star in the middle.
- The upper pattern is made out of dodecagons. They bear as well a 6-pointed star in the middle, but the orientation differs from the one in the pattern of nonagons.
Follow step by step in the applet below:
- Create e regular hexagon with doubled edges
- Create a tiling with these haxagons. Since the edges are doubled, they wil overlap, creating small hexagons in their vertices.
- Create a dodecagon with equal center as a hexagon.
- Do this for every hexagon to create overlapping dodecagons, creating 6-pointed stars in the centers.
- Create a light blue 6-pointed star, rotating a 6-pointed star in the center of a dodecagon.
- Do this in each dodecagon.
- Elongating the sides of three light blue stars you can create an irregular nonagon around a vertex of a hexagon.
- Do this for all vertices to create a decorative pattern of overlapping nonagons.